
- Видео 53
- Просмотров 6 397 342
commutant
Добавлен 3 апр 2011
High quality lectures on fundamental topics in mathematics.
partial differential equations
ordinary differential equations
fourier series
partial differential equations
ordinary differential equations
fourier series
Population modeling with differential equations
Link to Mathematica file:
drive.google.com/file/d/1_oU5jsYzrwoD2X-lUYlzyUUGl0zWRDFz/view?usp=sharing
drive.google.com/file/d/1_oU5jsYzrwoD2X-lUYlzyUUGl0zWRDFz/view?usp=sharing
Просмотров: 4 075
Видео
Introduction to ordinary differential equations and initial value problems
Просмотров 8123 года назад
We solve some differential equations by guessing and checking, then look at an example of an initial value problem.
ODE | Slope fields and isoclines example
Просмотров 47 тыс.6 лет назад
We give a brief example of sketching a slope field via two methods: plotting slopes at various points, and using isoclines.
Multivariable Calculus | Integration in polar coordinates
Просмотров 2,8 тыс.6 лет назад
An example of setting up an integral over a portion of an annulus in polar coordinates, including discussion of dA and the appearance of the factor of r.
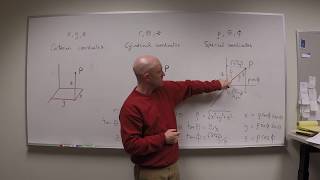
Multivariable Calculus | Polar, Cylindrical and Spherical coordinates
Просмотров 22 тыс.6 лет назад
First, a quick review of polar coordinates, including the conversion formulas between cartesian and polar. Next an introduction to the 3d coordinate systems of cylindrical and spherical coordinates.
ODE | Repeated eigenvalues explanation and example
Просмотров 28 тыс.6 лет назад
An example of a linear differential equation with a repeated eigenvalue. In this scenario, the typical solution technique does not work, and we explain how fix the problem.
Multivariable Calculus | Vector line integral intuition
Просмотров 1,2 тыс.6 лет назад
Four examples of line integrals over vector fields, with an intuitive discussion of what values to expect for them.
Multivariable Calculus | Scalar line integral example
Просмотров 7096 лет назад
An example of setting up and computing a scalar line integral. In this example we compute the mass of ring with a varying density.
ODE | Phase lines
Просмотров 20 тыс.6 лет назад
Introduction to the idea of a phase line. We construct a phase line for a logistic equation, starting with the slope field. Then we construct an equation for a given phase line.
Multivariable Calculus | Integration example in cartesian, cylindrical and spherical coordinates
Просмотров 1,4 тыс.6 лет назад
We set up an integral over a 3d shape (a solid cone) in all three popular 3d coordinate systems: cartesian, cylindrical and spherical.
Multivariable Calculus | Vector line integral example
Просмотров 5736 лет назад
An example of setting up and computing a vector line integral.
Multivariable Calculus | dV (volume element) in 3d coordinate systems
Просмотров 4,8 тыс.6 лет назад
We give a geometric explanation of dV (small element of volume) in Cartesian, cylindrical and spherical coordinates, including nice pictures.
Multivariable Calculus | Integrating over a portion of a half cylinder
Просмотров 4,8 тыс.6 лет назад
An example of an integral over a 3d shape (a portion of a half cylinder) in 3d cartesian coordinates.
PDE | Finite differences: introduction
Просмотров 236 тыс.11 лет назад
An introduction to partial differential equations. PDE playlist: ruclips.net/user/view_play_list?p=F6061160B55B0203 Topics: introduction to the idea of finite differences via an Euler's method example
ODE | Initial value problems for second order equations
Просмотров 86 тыс.12 лет назад
Examples and explanations for a course in ordinary differential equations. ODE playlist: ruclips.net/p/PLwIFHT1FWIUJYuP5y6YEM4WWrY4kEmIuS We define and solve an initial value problem for a second order linear differential equation, using solutions found earlier. We also discuss the idea of being able to solve *any* initial value problem.
ODE | Superposition principle example
Просмотров 79 тыс.12 лет назад
ODE | Superposition principle example
ODE | The logistic population model
Просмотров 25 тыс.12 лет назад
ODE | The logistic population model
ODE | Integrating factors general method
Просмотров 46 тыс.12 лет назад
ODE | Integrating factors general method
ODE | Integrating factors introduction
Просмотров 41 тыс.12 лет назад
ODE | Integrating factors introduction
ODE | Existence and uniqueness example
Просмотров 199 тыс.12 лет назад
ODE | Existence and uniqueness example








your 2 is just 6
What software are you all using, i am seriously curious to know. I also could have sworn i heard clicking, u using a mouse my dear friend?!?!? thats some talent up there. And really appreciate the explanation.
crystal clear explaination
wow, thanks for the vid.
Thanks for clearing it up! My college professor wasn’t clear of how to determine linear or nonlinear but this video is so helpful.
i have an exam in 2 days wish me luck
Love ❤❤❤❤
oh my!! The 3D graph finally made me understand. Thanks, commutant!
Awesome video! Neat handwriting!
Imagine heat from a candle. That heat is represented as a physical heat form.
Won't sleep my voxels aee shaky
thanks for the vid man.truly helpful
This is so helpful!! Thank you very much!! unbelievable good content
Thank you very much.understood it very well.❤❤❤❤
chmar salla
Thank you for this introduction!
What is the difference between f(x) and F(x) when talking about nomenclature?
Great video
Hey. This is exceptionally clear. Thank you!
Good Teacher ever I have seen in my life
very impressive lecture
Thank you
ma man that's amazing explination
what application were you using to write
I’m watching this as a seventh grader cause I’m bored
You are a great explainer. I dont know why you stopped!
You sir, have just saved me
This is the first time I have come across your channel. By watching this video on logistic equation example, I was able to solve an assignment step by step and get the correct answer. This makes sense. Thank you so much. I have already subscribed.
Thank you very much for making this video!!
thats a good lecture
1957 Lenny Rapids
The writing is too small please in your future videos please zoom
guys dont come to the future, math still sucks
incredible video
SPLENDID. Well-played.
How did you explain it in so simpler way.. Its amazing Thanks 👍
its really this simple
Excellent thank so much!
Bro conclude what my professor said in 1.5 hours in 3 min.
if there was a cosntant for example 1 the equality will break once you sum both of the scaled solutions.
current taking a year out and studying this on my own, he's very good
my professor glossed over this, the whole class forgot about it, and it showed up on the midterm. Everyone was completely stumped it was hilarious.
😂
thank you very much
Wow. The only video I was looking for to help me in answering the ODE 1 assignment. Thanks , big up man. 👊🇰🇪
This is very interesting.
so... why are u and v equal? The notation used in this video is absolutely horrible. u(x,t), is that u as a function of x and t, and v(ξ,t) v as a function of ξ and t? How is ∂u/∂t computated as described? Is u = u(x,t), so ∂u/∂t = ∂[v(ξ,t)]/∂t? Far too many steps being missed out, here.
Isn't 4:22 to be a total derivative on the rhs and partials on the lhs.
I think there is a h missing in the denominator of the definition of the derivative
where do we get our general sollution of EM wave? is it from the Helmholtz then find the solution of E (electric field)?